Puzzles and Riddles

Learning Goals:

  • Solve problems requiring the estimation and calculation of areas of rectangles
  • Multiply and divide whole numbers using a variety of tools and strategies
  • Determine the missing numbers in equations

The Challenge:

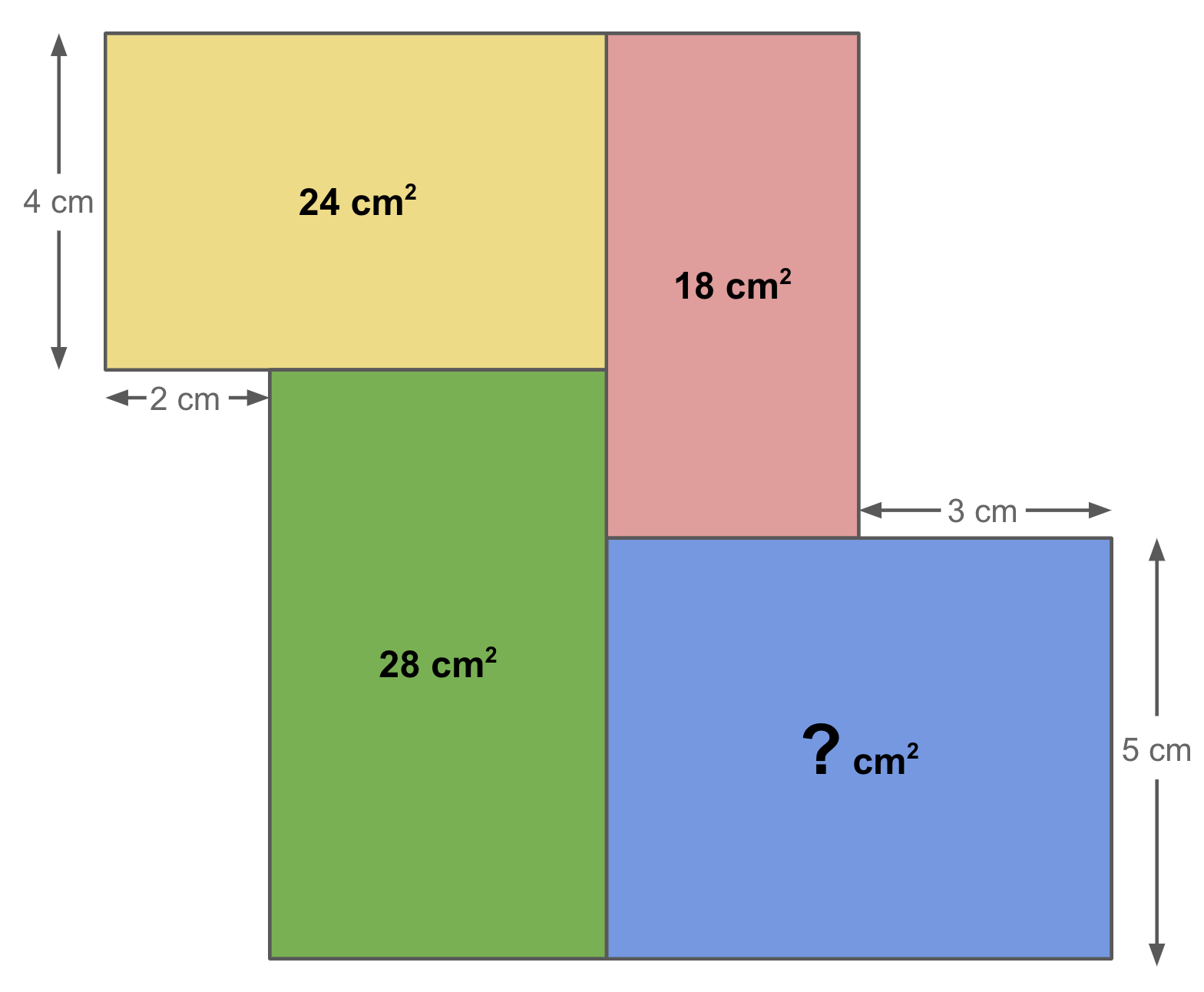
The goal of an area maze puzzle is to use what you know about the area of rectangles, multiplication, and division, to find unknown areas or dimensions (labelled with a question mark.) How could you use what you know in the puzzles below to find the mystery area of the blue rectangle OR the mystery length of the missing blue dimension?

Reminder:

The area of a rectangle can be found by multiplying the length x the width. These puzzles are not drawn to scale, so measuring side lengths with a ruler won’t help you solve them!

Two rectangles are connected to form a capital L shape. The yellow rectangle sits on top of the left side of the red rectangle, and has a labelled area of 30 square cm. Its left side is labelled with a length of 5 cm. The largest, red rectangle is on the bottom and has a labelled area of 33 square cm. Its top side that sticks out past the yellow rectangle is labelled as 5 cm long, and its right side has an unknown length, labelled with a question mark.

Four rectangles are connected to form a Z shape. The yellow rectangle in the top left corner, above the green rectangle and left of the red rectangle. It has a labelled area of 24 square cm, its left side is labelled with a length of 4 cm, and its bottom side that sticks out past the green rectangle is labelled with a length of 2 cm. The smallest, red rectangle is in the top left corner, above the blue rectangle and right of the yellow rectangle. It has a labelled area of 18 square cm, and none of its side lengths are labelled. The green rectangle is in the bottom left corner, underneath the yellow rectangle and right of the blue rectangle. It has a labelled area of 28 square cm, and none of its side lengths are labelled. The blue rectangle is in the bottom right corner, right of the green rectangle and below the red rectangle. Its unknown area is labelled with a question mark, its right side is labelled with a length of 5 cm, and its top side that sticks out past the red rectangle is labelled with a length of 3 cm.

A 4x4 grid of different sized grey rectangles, with one near the middle coloured blue. Some areas are labelled: In the top row, the first two rectangles are blank, and the last two are labelled 30 square cm and 40 square cm. In the second row, the first two rectangles are labelled 36 square cm and 28 square cm, and the last two are blank. In the third row, the first rectangle is blank, the second is the blue rectangle labelled with a question mark, the third is blank, and the last is labelled 48 square cm. The bottom row has the first rectangle labelled 63 square cm, the second rectangle is blank, the third is labelled 42 square cm, and the last is blank. The only length labelled in the grid is the left hand side of the bottom row, which is 7 cm long.

Questions and Prompts to Support your Child:

  • What have you tried so far? What could we try together?
  • What missing dimensions do we need to calculate the area of the blue rectangle? How can we use partial dimensions to help us progress? (hint: think addition/subtraction!)
  • If you feel stuck, it might help to start by looking for known dimensions – if we know that this 30 cm2 rectangle has a width of 5 cm, what would the length need to be? How does this newfound dimension help us get closer to finding our mystery area?

Extensions & Adaptations:

  • If calculations are feeling too tricky, grab a calculator!
  • This might be our first time experiencing a known area as a means to figure out a missing dimension – remember that division is a way to “undo” multiplication!
  • Try creating your own area puzzles by sketching some connected rectangles, labelling all of the lengths and widths, calculating the areas, then erasing all but a few so someone can solve it. How much information do you need to leave so it’s still possible to solve? How much information is too much and would make it too easy? Is there a way to include partial dimensions to increase the difficulty?
  • Check out areamaze.com for many more puzzles – including some more challenging options!

Adapted from Areamaze.com & Puzzleoftheweek.com